
Hopf Fibration (https://nilesjohnson.net/hopf.html)

Mathematics has had a profound effect in shaping modern art: not in its ability to generate attractive patterns but in exposing the artists to spatial possibilities not easily observed in their physical world. One such spatial possibility is the fourth dimension, which is, for the most part, inaccessible to human perception.
The question of whether or not this invisible extension of reality even exists was keen in the minds of many in the early decades of the 20th century. Fueling the question was the popularity of occultism, spiritualism and other esoteric sciences at the turn of the century. Since these belief systems posited the physical existence of spirits, they needed a physical space to locate them. Many early abstract artists subscribed to theosophy and believed in an expanded reality in order to house the occult.
Other more analytical artists were influenced by the growth of visual models of advanced mathematics that burgeoned during the late 19th century. Many of these models were predicated on geometries that required the invention of higher dimensions in order to be. Geometers discovered that many of these forms which seemed to be discrete were actually portions of the same four dimensional object. Einstein’s ground-breaking theories further popularized interest in higher dimensions and alternate geometries. His theories assumed such geometries, which offered spaces very different from that offered by everyday experience.
Barbara Hepworth’s spherical sculptures appear to be an example of exposure to such visual models. They bear a remarkable resemblance to 3D visualizations of the hypersphere, the four dimensional analog of the sphere. Like most of her geometric knowledge these were likely introduced to her by her good friend J.D. Bernal, known to science as the founder of molecular biology.
Bernal’s broad range of knowledge earned him the sobriquet ‘Sage’ and stretched from political activism to art. His friendship with artists such as Naum Gabo, Henry Moore, Ben Nicholson, Barbara Hepworth and others garnered him inclusion in the publication of “Circle”, an exposition by and of the major constructivist artists in England and Europe. He regarded artistic enterprise as being in accord with science, especially since both strive for the ‘best’ form. Drawn to write about the arts and architecture, Bernal penned the introduction to Barbara Hepworth’s first exhibition catalogue in 1937, where he referred to her introduction of a fourth dimension as “a closed curve in time.” Coincidentally Hepworth began tentative explorations in plaster of spherical formats shortly after this exhibit, but she exhibited none until six years later.
The exhibit summarized a body of work begun after she gave birth to triplets. Following this hiatus her sculptures turned from concerns with the human figure and began embracing geometry. At this time Bernal met Hepworth through her friend and his paramour Margaret Gardiner, the artist and collector. This began a long series of visits to Hepworth’s St.Ives studio where Bernal discussed her work from the lens of the geometer and brought sample images of his x-ray crystallography. These latter stimulated a set of remarkable drawings. The drawings were not depictions, but parallels of mathematics: they were to function only as art. Despite their avid interest in science, Hepworth and others of her artistic circle were inclined to keep art and science on separate though parallel paths. Naum Gabo, perhaps the most scientifically sophisticated of the circle (he was thought to be knowledgeable of quantum physics), stated their position in a 1957 interview:
“Art and Science are two different streams which rise from the same creative force and flow into the same ocean of the common culture, but the currents of these two streams flow in different directions.”
Although Gabo’s statement agrees with Bernal’s view, Gabo himself shunned Bernal. Having lived in Soviet Russia, Gabo was put off by the scientist’s Communist activism.
Many artists of the early 20th century maintained an ambivalence toward mathematics and science even when inspired by contact with these fields. Kasimir Malevich, for example, created visualizations of four dimensions on at least two separate occasions using separate approaches. His visualizations were premised on classical geometry, but he never revealed just how he used that geometry. Inspired by Nikolai Lobachevsky’s notion of pangeometry, which held that an unknown multitude of geometries are possible, Malevich believed that one such geometry could be developed specifically for art. Other of Malevich’s Russian contemporaries openly expressed the importance of mathematics to the new art. Alexander Rodchenko, for example, averred that art was actually a field of mathematics.
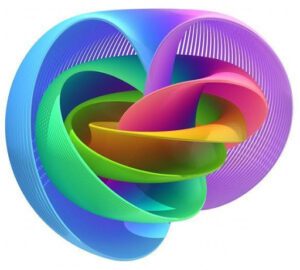
The evidence that Hepworth’s exposure to geometry extended to the fourth dimension is circumstantial, although compelling. Such exposure would surely have come from Bernal. Bernal well understood mathematical descriptions of the fourth dimension. As a student he introduced the use of quaternions, a system for computing in four dimensions, to calculate transformations of crystals. Consequently, when the noted mathematician Heinz Hopf used quaternions to construct his projections of the hypersphere, Bernal could well have understood Hopf’s method. Hopf’s 1931 discovery, known as the Hopf fibration, occurred just prior to Bernal’s contact with Hepworth.
It is doubtful that Hepworth’s goal was higher dimensional visualization or that she even comprehended the fourth dimension. Her intent was clearly sculptural: the conflation of interior and exterior spaces as presented in Hopf’s model.
One aspect of four dimensional objects is that inside and outside do not exist as we know it. In fact three dimensional projections of four dimensional objects when rotated within the fourth dimension appear to turn inside out when projected as a three dimensional object. This is likely what intrigued Hepworth: sculptures that interpreted the sphere by simultaneously displaying both the inside and outside and then linking both into one form.
Most of Hepworth’s spherical and oval sculptures from the early 40s to the early 70s often realized this link by relying to some degree on the inner space spiraling out to become the exterior. In this regard most depictions of the Hopf fibration also incorporate three dimensional spirals. Hopf calculated his model as a series of tightly packed circular fibers dispersed within a normal sphere by using quaternions. The close-packed series of circles coiled and expanded to become the enclosing sphere.
Just as with three-dimensional objects projected into two dimensions, the apparent configuration of a four dimensional object projected into three dimensions can vary greatly depending on one’s point of view and the position of the object in four dimensional space. Many of Hepworth’s spherical sculptures seem to replicate projections of the hypersphere from alternate views derived by subsequent mathematicians. Without seeing any of these alternate projections, Hepworth’s sculptural experiments had coincidentally integrated interior and exterior in manners similar to those arrived at mathematically.
It seems that Hepworth’s striving for optimum aesthetic forms paralleled, as Bernal believed, the mathematicians’ own efforts.
Stephen Luecking
Volume 34 no 4 March/April 2020 pp 13-14

